THE MOLE CONCEPT AND RELATED CALCULATIONS.

The Mole as a Unit of Measurement

The mole is the unit of amount in chemistry.  It provides a bridge between the atom and the macroscopic amounts of material that we work with in the laboratory.  It allows the chemist to weigh out amounts of two substances, say iron and sulfur, such that equal numbers of atoms of iron and sulfur are obtained.  A mole of a substance is defined as:
The mass of substance containing the same number of fundamental units as there are atoms in exactly 12.000 g of ¹²C.

Fundamental units may be atoms, molecules, or formula units, depending on the substance concerned. At present, our best estimate of the number of atoms in 12.000 g of ¹²C is 6.022 x 10²³, a huge number of atoms.  This is obviously a very important quantity.  For historical reasons, it is called Avogadro's Number, and is given the symbol N_A. (Amedeo Avogadro honored himself)
 6.0 × 10²³. The value 6.0 × 10²³ is called Avogadro’s constant or Avogadro’s number and is abbreviated as N_A or L .It is named after the nineteenth-century Italian chemist, Amedeo Avogadro.

The value 6.0 × 10²³ is obtained through the following relationship.The mass of one atom of carbon-12 is 1.993 × 10^-23g. Then, the number of atoms present in 12g of carbon-12 is derived as follows:

1 atom = 1.993 × 10^-23g

X atoms = 12g

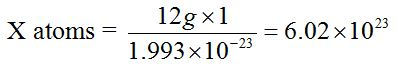
\ X = 6.0 × 10²³ atoms.

Therefore, the number of atoms in 12g of carbon-12 and hence the number of particles in a mole are 6.02 × 10²³ atoms.

Hence, Avogadro’s number is the number of atoms in exactly 12g of carbon-12 isotope.One mole of any substance contains as many as many elementary particles as the Avogadro’s number (constant).

So, from the above explanation, the mole can be defined as the amount of a substance that contains as many elementary particles as the number of atoms present in 12g of carbon-12 isotope.

Substance	Formula	Relative formula mass, Mr	Mass of one mole (molar mass)	This mass (1 mole) contains
Carbon	C	12	12g	6.0 × 1023 carbon atoms
Iron	Fe	56	56g	6.0 × 1023 iron atoms
Hydrogen	H2	2 × 1 = 2	2g	6.0 × 1023 molecules
Oxygen	O2	2 × 16 = 32	32	6.0 × 1023 molecules
Water	H2O	(2×1) + 16 = 18	18g	6.0 × 1023 formula units
Magnesium oxide	MgO	24 + 16 = 40	40g	6.0 × 1023 formula units
Calcium carbonate	CaCO3	40+12+(3×16) = 100	100g	6.0 × 1023 formula units
Silicon oxide	SiO2	28 + (2 × 16) = 60	60g	6.0 × 1023 formula units
Fe3+	Fe3+	56	56g	6.0 × 1023 iron(III) ions
Cl-	Cl-	35.5	35.5g	6.0 × 1023 chloride ions
e-	e-	-	-	6.0 × 1023 electrons

The other substances, which also exist as molecules, include ozone molecule (gas), O₃; phosphorus molecule (solid), P₄; sulphur molecule, S₈, etc.

In real life, when dealing with large numbers of small objects, it is usual to count them in groups. The objects are grouped and counted in unit amounts. For example, we buy a carton of soap, a gallon of kerosene, a crate of soda, a dozen of pencils, a ream of papers, etc.

Some units of measurement

Unit	Number of objects per unit
Pair	1 pair = 2 objects, e.g. gloves, shoes, socks, scissors, etc are always sold in pairs.
Dozen	1 dozen = 12 objects e.g. a dozen of cups, plates, spoons, etc.
Gross	1 gross = 144 objects, e.g. a box of blackboard chalk contains 144 pieces of chalk.
Ream	1 ream = 500 objects, e.g. papers are sold in reams of 500 sheets.
Mole	1 mole = 6.02 ×1023 particles. In chemistry, extremely small particles are expressed in moles. For example:1 mole of atoms = 6.02 ×1023 atoms1 mole of electrons = 6.02 ×1023 electrons1 mole of protons = 6.02 ×1023 protons1 mole of ions = 6.02 ×1023 ions1 mole of molecules = 6.02 ×1023 molecules

Molar Quantities of Different Substances

The mass of one mole of any substance (its molecular mass) is the atomic mass or molecular mass expressed in grams (or kilograms). For convenience, chemists prefer to express mass in grams, although the SI unit of mass is the kilogram. This is because the amount of substances which chemists usually work with in science laboratories, is quite small and if their masses are expressed in kilograms, the numbers used would be extremely small.

You can calculate the molar mass (M) of any substance by summing up the relative atomic weights of its constituents atoms. For example, ethanol, C₂H₅OH, contains two carbon atoms, six hydrogen atoms and one oxygen atom. So, the molar mass of ethanol can be calculated thus: Molar mass of C₂H₅OH = (2 × 12) + (6×1) + 16 = 46g.

In a similar way, molar masses of other compounds can be calculated. For example, the molar mass of sodium chloride, NaCl, is calculated by adding together the relative atomic masses of the constituents elements (Na = 23 and Cl = 35.5) = 23 + 35 = 58.5g (g mol^-1).

It is important to note that relative atomic mass or relative molecular mass has no unit while molar masses are always expressed in grams or kilograms.

The molar mass of a compound is the same as the relative molecular mass and the molar mass of an element is the same as the relative atomic mass (A_r) of that element. The only difference lies in the units.

Example 1

• M(CO₂) = 44g (or g mol^-1) = molar mass of carbon dioxide
• M_r(CO₂) = 44 = relative molecular mass of carbon dioxide
• M(Fe) = 56g (or g mol^-1) molar mass of iron
• M_r(Fe) = 56 = Relative atomic mass of iron

Similarly, the molar masses of each of the following substances can be calculated using values for the relative atomic masses of the elements.

Molar masses of different substances

Substance	Formula	Molar mass
Ammonia	NH3	14 + 1×3 = 17g
Ammonium chloride	NH4Cl	14 + (1×4) + 35.5 = 53.5g
Lead (II) nitrate	Pb(NO3)2	207 + (14×2) + (16×6) = 331g
Sulphuric acid	H2SO4	(1×2) + 32 + (16×4) = 98g
Calcium carbonate	CaCO3	40 + 12 + (16×3) = 100g
Potassium dichromate	K2Cr2O7	(39×2) + (52 ×2) + (16×7) = 294g

Application of the Mole Concept

Known Masses of Elements, Molecules or Ions to Moles

Convert known masses of elements, molecules or ions to moles

In experimental work, chemists work with varying masses. They cannot always use one mole of a substance. The equation that links the mass of a substance to the number of moles present is:

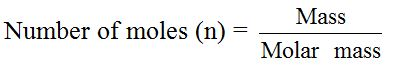
Example 2

Convert 49g of sulphuric acid, H₂SO₄, into moles.Given:Mass = 49g; molar mass = 98g

Formula:

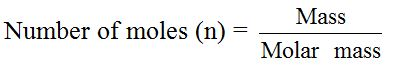
Solution:49g of H₂SO₄= 49/98= 0.5 mol.

Known Volumes of Gases at S.T.P to Moles

Convert known volumes of gases at S.T.P to moles

The volume occupied by one mole of a gas at standard condition of temperature and pressure has been scientifically determined, and it is found to be 22.4 dm³. This volume is called the molar volume of a gas. The molar volume of a gas, therefore, has the value of 22.4 dm³ at s.t.p. Remember that 1 dm³ (1 litre) = 1000 cm³. One important thing about this value is that it applies to all gases. Therefore, at s.t.p. 32g of oxygen (O₂) or 17g of ammonia (NH₃) or 44g of carbon dioxide (CO₂) or 40g of argon (Ar) will occupy a volume of 22.4 dm³. This makes it easy to convert the volume of any gas at s.t.p. into moles, or moles into volume. However, it is important to note that as the conditions of temperature and pressure change the molar volume will also change.

The number of moles of a given sample of gas is obtained by dividing the volume of the gas by molar volume (22.4 dm³).

For example, 4.4d m³ of carbon dioxide gas at s.t.p. = 4.4/22.4= 0.196 mol.Similarly, 2.24 dm³ of neon gas at s.t.p. = 2.24/22.4= 0.1 mol.

If the volume of the gas is given in cm³, then it should be divided by the molar volume of a gas expressed in cm³. For example, 560 cm³ of nitrogen gas = 560cm³/22400cm³ mol= 0.025 mol.

Alternatively, the volume may, first, be converted to dm³ and then divides by the molar volume, expressed in dm³, that is, 0.46dm³/22.4dm³ = 0.25mol

Masses of Solids or Volumes of Known Gases to Actual Number of Parties

Change masses of solids or volumes of known gases to actual number of parties

The number of particles in one mole of any substance is 6.02 × 10²³. To find the number of particles in a substance, we use the expression:

• N = n.L, where
• N = the number of particles in that substance;
• n = the amount of substance (moles); and
• L = the Avogadro’s constant (6.02 × 10²³).

This conversion requires two steps: first convert the mass of solid or volume of gas to moles, and then multiply the number of moles by the Avogadro’s constant. For example, to convert 5.6 dm³ of ammonia gas to the actual number of ammonia (NH₃) molecules, change 5.6 dm³ of ammonia to moles =0.46dm³/22.4dm³=0.25 mol. Then multiply by the Avogadro’s constant to get the total number of molecules0.25 × 6.02 × 10²³ = 1.5 × 10²³ molecules

Similarly, 1.12 dm³ of hydrogen gas = 1.12/22.4= 0.05 mol. This is equal to 0.05 × 6.02 × 10²³ = 3.0 ×10²² molecules

Alternatively, we may find out the number of particles by converting the given volume to the number of molecules straight forward without passing through the number of moles first. We know that one mole (22.4 dm³) of a gas at s.t.p. = 6.02 × 10²³ molecules. So, 5.6 dm³ = 5.6×6.02 × 10²³/22.4= 1.5 × 10²³ molecules

Molar Solutions of Various Soluble Substances

A molar solution is a solution which contains one of the compound in one litre (1 dm³ or 1000 cm³) of the solution.Let us consider the case of sodium hydroxide, NaOH. The molecular weight of this compound is 40g. Therefore, a molar solution of sodium hydroxide will contain 40g in 1000 cm³(1 dm³) of the solution.

Also, consider anhydrous sodium carbonate, Na₂CO₃. 1 mole of this carbonate weights 106g. Hence, its molar solution will contain 106g of the anhydrous salt in 1000 cm³ of solution.If, however, 0.1 moles (10.6g) of the solute is dissolved in 1.0 dm³, the solution is 0.1 molar. But if 0.1 moles is dissolved in 0.1 dm³ of the solution, the solution is still 1.0 molar (since 1 dm³ of solution would contain 1.0 mole of the solute).

The molecular weights of some common substances are shown below:

Compound	Molecular weight (1 mole)
Potassium hydroxide, NaOH	56g
Hydrochloric acid, HCl	36.5g
Sulphuric acid, H2SO4	98g
Sodium chloride, NaCl	58.5g
Sodium bicarbonate, NaHCO3	84g
Calcium hydroxide, Ca(OH)2	74g

The molar solution of each of these substances can be prepared by dissolving one mole of each substance in 1000 cm³ (1 dm³) of distilled water. We see, therefore, that 40g of sodium hydroxide in 1000 cm³ of solution will give a 1.0M solution. Hence, 20g of the hydroxide should give a 0.5M solution. In a similar way, we can make derivative solution concentrations ranging as follows: 0.1M, 0.2M, 0.3M, 0.4M….1M, 2M, etc.

However, in each case the amount of solution should always be 1000 cm³. The concentration ranges like these are known as molarities of solutions. Hence, 0.5M sodium carbonate can also be read as “a sodium carbonate solution with a molarity of 0.5M.”

The Concentration of Solutions

When a chemical substance (the solute) is dissolved in a given volume of solvent, we can measure the “quantity” of solute in two ways; we can measure either its mass (in grams) or its amount (in moles). The final volume of the solution is usually measured in dm³.

When we measure the mass of the solute in grams, we obtainthe mass concentration in g/dm³

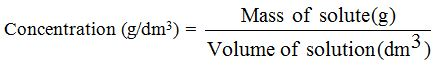
Example 3

Calculate the concentration (g/dm³) of sodium chloride solution (NaCl) that contains 20g of sodium chloride in a final solution of 100 cm³

Solution

First, convert the given volume to dm³

Volume (dm³) = 100/1000= 0.1 dm³

Then, work out the concentration of the solution by dividing the mass (weight) of solute (g) by the volume (dm³).

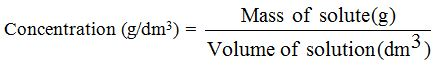
=20g/0.1dm³

= 200g/dm³

Alternatively, we could calculate the concentration straightforward without having to convert the given volume into dm³, e.g.:If 20g of the solution are contained in 100 cm³ of the solution, then the amount of solute in 1000 cm³ (1 dm³) of the solution would be

1000×20/100 = 200g/dm³

Calculations Based on the Mole Concept

A chemist always wants to know how much of one substance would react with a given amount of another substance. This is achieved by use of balanced chemical equations. Such equations are called stoichiometric equations.

A stoichiometric equation is the one in which the reactants and the products are correctly balanced; all the atoms, ions and electrons are conserved. Such an equation gives correct mole ratios of reactants and products in chemical reactions. This quantitative relationship is called stoichiometry.

Consider an equation for the reaction between hydrogen and nitrogen to produce ammonia:

3H_2(g) + N_2(g)→ 2NH₃

This can be read as follows:

three moles of hydrogen reacts with one mole of nitrogen to yield two moles of ammonia.

The numbers 3, 1 and 2 are called stoichiometric coefficients. They tell us the proportions in which the substances react and in which the products are formed.

Example 4

What volume of carbon dioxide (CO₂) measured at s.t.p. will be produced when 21.0g of sodium hydrogencarbonate (NaHCO₃) is completely decomposed according to the equation.2NaHCO_3(s)→ Na₂CO_3(s) + CO_2(g) + H₂O_(l)

Solution

First, find the weight of carbon dioxide that will be produced by the hydrogencarbonate.

• Mass of 2NaHCO₃ = 2 × 84 = 168g
• Mass of CO₂ = 44g

The weight of carbon dioxide produced can be obtained from the following relation:

168g ≡ 44g

21g ≡ X

X = 21×44/168 = 5.5g

The weight of carbon dioxide produced = 5.5g

Then, convert this weight of CO₂ to volume at s.t.p.We know that one mole (44g) of carbon dioxide at s.t.p. occupies 22.4 dm³

That is, 44g ≡ 22.4dm³

5.5g ≡ X dm³

X = 5.5×22.4/44 = 2.8dm³